More moment of inertia, and torque

Today, we will cover two topics.

First, we'll learn two methods for computing the moment of inertia of objects which are more complicated than very simple shapes made of uniform materials.

After that, we'll start to discuss the rotational analog of Newton's Second Law. How can we move F = ma into the rotational realm? By defining a rotational equivalent to force: torque.

Computing moments of inertia

Problems involving rotational motion often require one to know the moment of inertia of objects. Now, under certain simple circumstances, we know how to compute it:

if the object is a few discrete point masses, just use

if the object is a simple shape, of UNIFORM density, and rotates around its center, look up the moment of inertia in this table of moments of inertia (PDF) or this copy of the table in PNG format.

if the object is an extended, continuous body with a UNIFORM density, it might be possible to integrate over all the little pieces in the body.

But what if the situation is not quite so simple? For example, suppose that the object IS a basic shape, but is NOT rotating around its center?

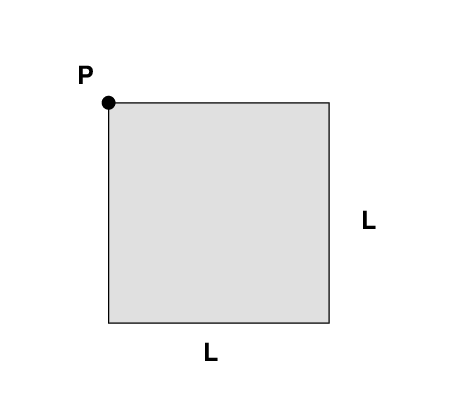
In this case, you can use the

parallel-axis theorem together with table of moments of inertia

Finally, what if the object in question is just not simple at all, but made of a material which changes in density from one location to another. In that case, we need to integrate around the rotation axis -- but take care to account for the mass of each section properly.

Integrating an object with changing density

The rotational analog of force: torque

You've seen the rotational analog of simple 1-D kinematics, which answer questions like

"how fast is it spinning?"
"when will it stop?"
"how many revolutions will it make in the next 29 seconds?"

But what about rotational dynamics, which addresses deeper questions, such as

"WHY is it spinning faster and faster?"
"what must I do to make the wheel slow down?"

Once again, we'll use your knowledge of physics in the linear world to guide us through the rotational realm.

Looking at the variables involved in Newton's Second Law in rotation: torque

A torque, it turns out, is a bit more complicated than a force; in order to compute the torque on an object, you need to know not only the size of the force involved, and the location at which it acts, but also the DIRECTION of the force.

How the direction of a force affects its torque

Stop the Earth!