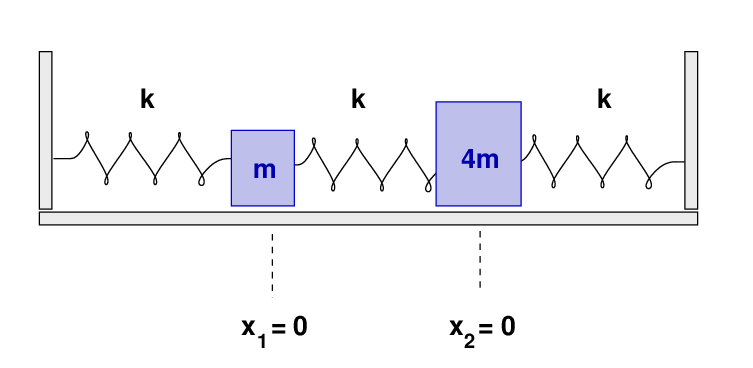
- What are the frequencies of the normal modes of this system? Call the lower frequency ω1 and the higher frequency ω2.
- What are the eigenvectors corresponding to each frequency?
- What are the values of the coefficients A1, A2, φ1, φ2, in the equations for the normal modes?
- Write an equation giving the position of block 1 (the 2 kg mass) as a function of time.
There will be two normal modes of oscillation, which can be described as
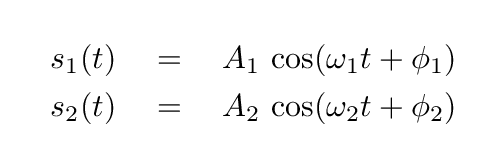
Suppose that, at time t = 0, the blocks are set so that x1 = 2 m , x2 = 0 m , v1 = 0 m/s , v2 = 0 m/s .
Answers to problem 1:
An ordinary molecule of carbon monoxide is made up of ordinary carbon and oxygen atoms:
mass of ordinary carbon mC = 12 mH
mass of ordinary oxygen mO = 16 mH
where
mass of ordinary hydrogen mH = 1.67 x 10-27 kg
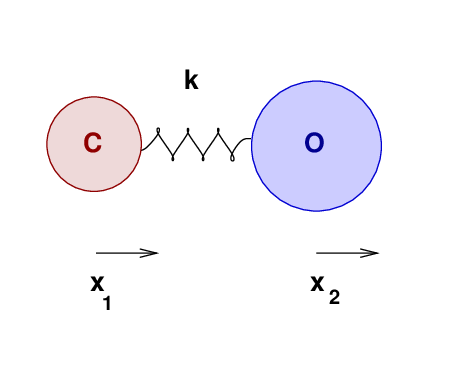
The force constant of the bond between these atoms is k = 1860 N/m. Suppose that these two atoms vibrate in their first normal mode.
- What is the angular frequency ω of this oscillation?
- If a vibrating molecule emits all its energy as an infrared photon, what will the wavelength of that photon be?
- What is the angular frequency ω of a "heavy" CO molecule in its first normal mode?
- If a vibrating "heavy" CO molecule emits all its energy as an infrared photon, what will the wavelength of that photon be?
A very small fraction, about 0.2 percent, of all oxygen atoms have two extra neutrons in their nucleus. These "heavy" oxygen atoms, called 18O atoms, have a mass which is 18 times mH. Suppose that one such "heavy" oxygen atom joins with an ordinary carbon atom to create a "heavy" carbon monoxide molecule, and suppose further than the force constant of the bond remains unchanged.
Answers to problem 2: