Practice some simple aperture photometry

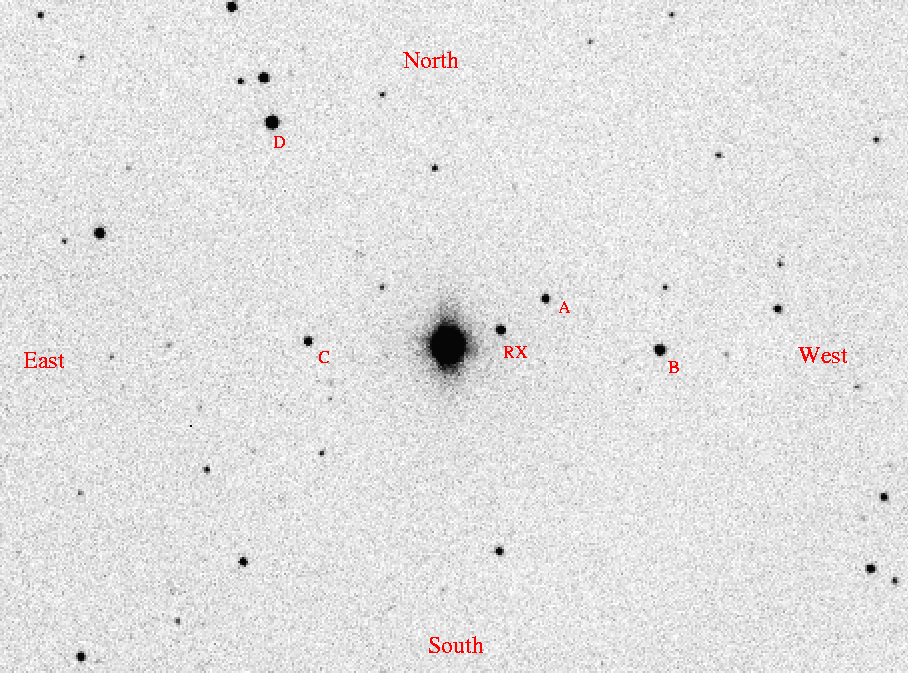
Let's practice some aperture photometry. We'll look at pictures of a field which has a very bright star near the center. Don't bother with it; instead, measure the fainter stars around it marked A, B, C and RX. The star marked RX is formally known as "RX J1643.7+3402"; you can look up information about it by visiting SIMBAD .

Each pair of astronomers should pick four of the images from the list below. To download an image, right-click on it and save to the desktop. Then read it into the MIRA program to measure instrumental magnitudes of the four stars.

Look here for the list of images. They cover the period 2002 Apr 12 UT 04:05:15 to 04:24:12, taken at roughly 36-second intervals. That corresponds to JD 2,452,376.67031 to 2,452,376.68347. For each of your images,
- right-click on the image name and select "Save Target As...";
- choose the Desktop as the place to put the local copies
A brief guide to using MIRA to do aperture photometry

Write down the instrumental magnitudes for each of the stars in each of the images, making a table like this:

                         Raw magnitudes
      Image         A         B        C        RX
   --------------------------------------------------
      rxa.040     -7.21     -8.20    -7.26    -7.61
      rxa.041     
      rxa.042     
      rxa.043

Next, create a table of differential magnitudes by subtracting the magnitude of star B from each of the other stars:

                      Differential magnitudes
      Image       (A-B)     (B-B)    (C-B)    (RX-B)
   --------------------------------------------------
      rxa.040      0.99      0.00     0.94     0.59
      rxa.041     
      rxa.042     
      rxa.043

When you are finished, write your differential magnitudes in the space provided on the blackboard.

The resulting differential photometry

Based on the measurements made by the workshop participants (each of whom measured 4 of the images), we derived differential light curves like this:

our differential measurements

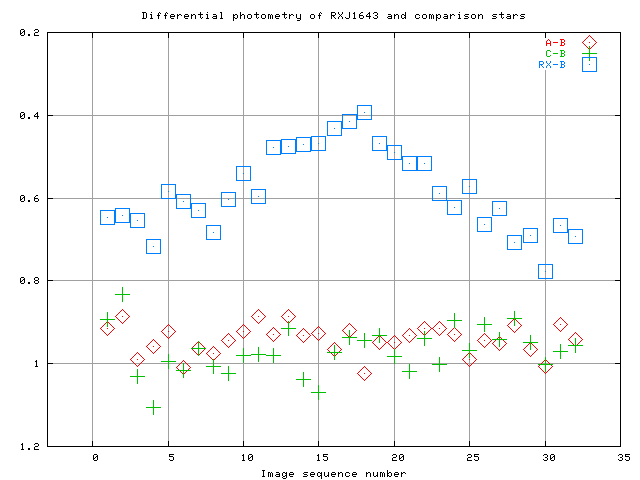
You can see that

star A is about 0.9 magnitudes fainter than star B, and remains at that level during the entire run
star C is about 1.0 magnitudes fainter than star B, and remains at that level during the entire run
the variable star RX ranges from 0.8 to 0.4 magnitudes fainter than star B, growing brighter and then fainter over the observing run (about 2.5 hours)

When I reduced the data myself, back in 2002, I found the same behavior; only the details a bit different.

my report on photometry of RXJ 1643 on Apr 11, 2002