Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
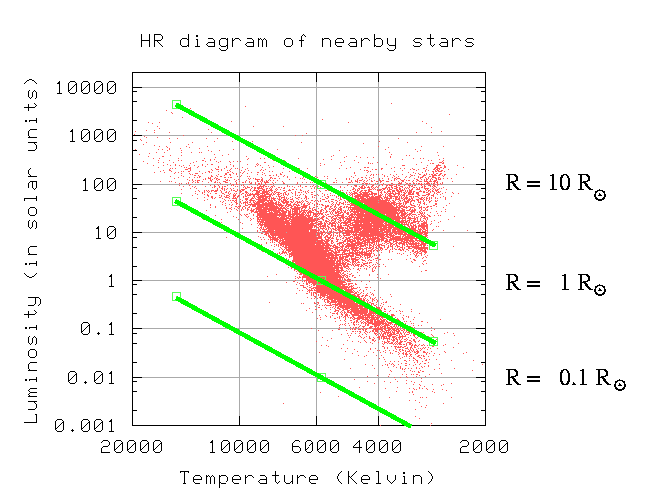
Most stars fall along a straight line in the HR diagram, running from upper-left to lower-right. That tells us that most stars must fall within a relatively narrow range of sizes.
But is there any way we can measure the size of a star more directly? After all, real stellar spectra aren't exactly like those of blackbodies, so these rough estimates might be wrong.
It turns out that there are several different methods for measuring the size of a star.
As we discuss each of the methods below, keep in your mind a single, concrete example: could this method determine the size of an ordinary star like the Sun, if it happened to be a mere 10 parsecs away from us?
Q: What is the angular size of a sun-like
star at a distance of 10 pc?
Express your answer in radians and
in milli-arcseconds (mas).
It seems obvious: if you want to measure the size of a star, just point your telescope at it and take a picture. Measure the angular size of the star in the image, then multiply by the distance to find the true linear diameter. What's so hard about that?
The problem is a phenomenon called diffraction. If you use a real optical system with an aperture of diameter D, light rays won't come to a perfect point at the focus; instead, interference from rays entering the aperture at different locations will form a fuzzy blob of light, surrounded by a series of faint rings. The image below was taken by a telescope with a mirror made of liquid mercury, 1.5 meters in diameter.
This pattern is called the "Airy pattern" after George Airy, Astronomer Royal of England, who first derived the angular size of the central blob and the rings which surround it. The intensity and spacing of the rings are related to the Bessell function of first order, J1. For our purposes, the important item is the angular size of the central blob, from its center to the first minimum in the diffraction pattern.
Q: In order to resolve a sun-like star at 10 pc,
using visible light (5500 Angstroms),
how large would a telescope's aperture have
to be?
It helps to work as far into the ultraviolet as possible, since the decrease in wavelength λ shrinks the Airy pattern. Unfortunately, the Earth's atmosphere prevents ultraviolet light from reaching the ground. Astronomers have used telescopes in space to take near-UV images of a very few stars in hopes of resolving them. In at least one case, they have succeeded -- barely. Here's an image from the press release:
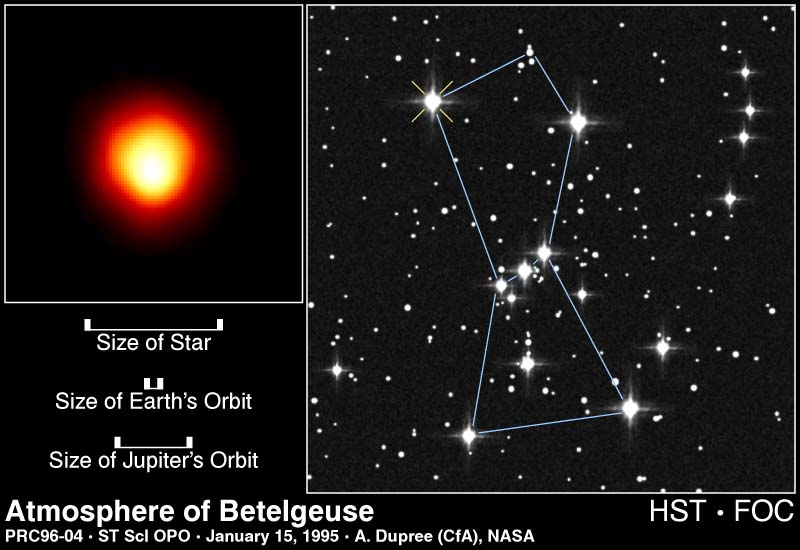
And here are a pair of radial profiles, of Betelgeuse and an unresolved star, from a conference proceeding describing the observations.
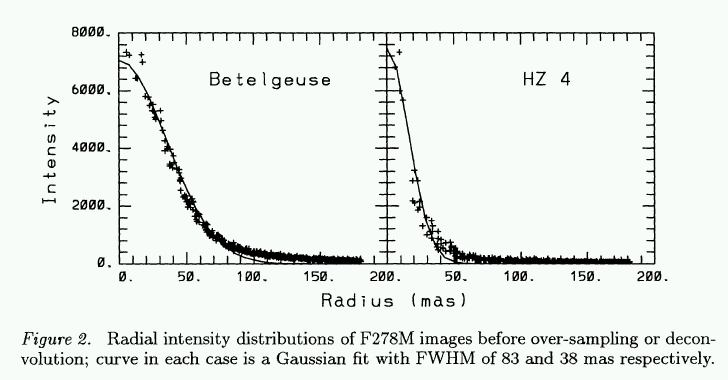
Q: The parallax of Betelgeuse is 0.00764 arcsec.
What is the diameter of Betelgeuse? Express
your answers in terms of the solar diameter.
The basic idea is simple:
Sounds easy, right? Just watch as the moon's limb covers the star:
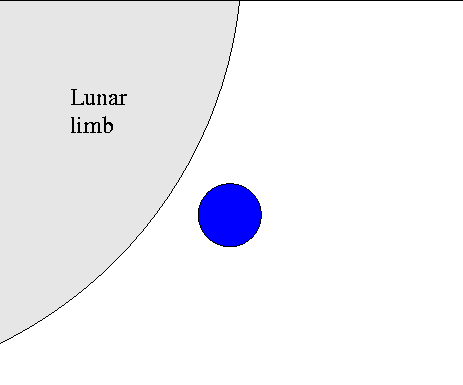
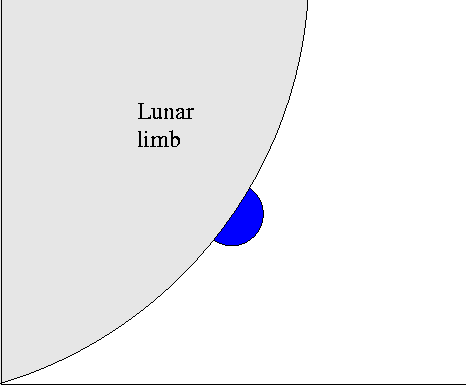
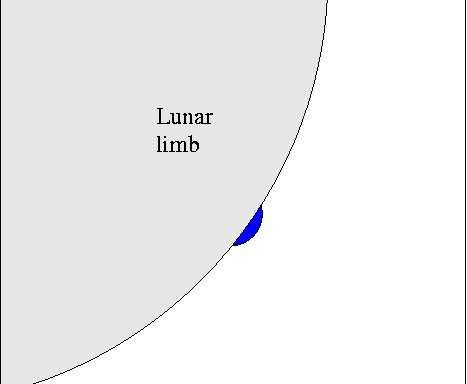
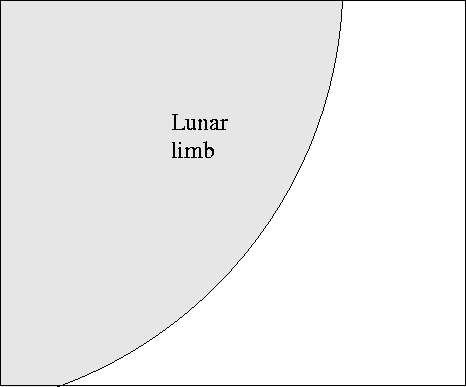
The time it takes for the star's light to disappear, Δt,
is just the angular size of the star θ divided by the angular speed v of the Moon through the sky.
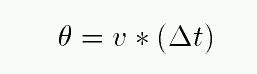
Q: What is the Moon's angular speed through the sky?
Express your answer in arcseconds per second.
Q: How long would it take for the Moon's limb to
cover a star of angular diameter 100 mas?
Uh-oh. It appears that any lunar occultation will be a very quick event. One will need
This requirement of collecting lots of photons in a very short time is a killer. The lunar occultation method is therefore restricted to relatively bright stars. It's also restricted to stars which happen to lie near the ecliptic, of course.
But ... it's even worse! It turns out that diffraction makes life difficult for astronomers again. As the Moon's limb begins to pass in front of the star's disk, it diffracts the light from the star. As an analogy, you know the diffraction pattern of light passing through a narrow slit.
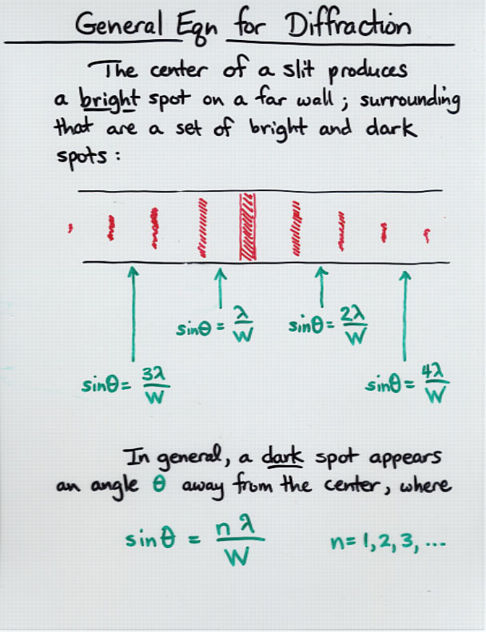
As the Moon's limb approaches and covers a star, we on the Earth see something like this pattern of alternating dark and light spots moving past our detectors. So the light curves (intensity versus time) that we record will be somewhat more complicated than the simple decreasing curve shown earlier.
The mathematical analysis of these real light curves is a complicated business. Once again, Bessel functions come into play. One must also make some assumptions about the star itself: is it a uniformly bright disk with a sharp edge, or is it limb-darkened like the Sun?
The bottom line is that the lunar occultation method is effective at measuring the angular diameters of stars down to about 1 mas. Back in 1987, White and Feierman published a catalog angular diameters of 124 stars measured by lunar occultations. A paper by Richichi states that another 50 stars were measured in this way up to 1998. The lunar occultation method has very clear limits, but clearly has given us a great deal of information on stellar sizes.
Fortunately, the Moon isn't the only "moving knife edge" we can use to determine the sizes of stars. There are many instances in which we can use one star as the "moving knife edge" to measure the size of a second star. All we have to do is find an eclipsing binary system: a pair of stars orbiting around each other, oriented in space so that one star passes in front of the other as seen from the Earth. If we measure the light coming from such a system carefully, we can detect the decrease in total intensity as a portion of one of the stars is covered:
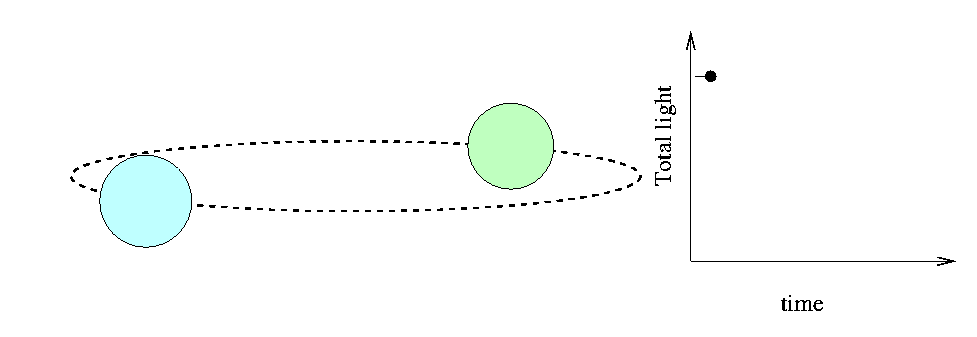
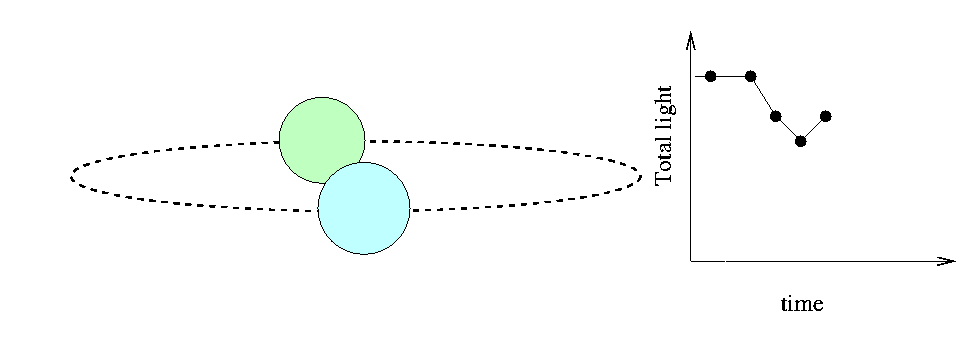
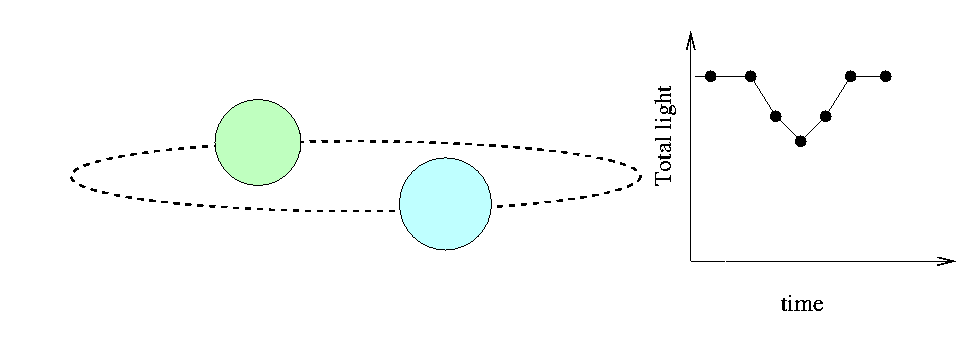
The time interval T between first contact and mid-eclipse depends on the sizes of the two stars and their relative velocity, (VA - VB). There are some complications, of course,
Wait a minute -- we need to know the orbital speeds of the two stars, VA and VB. How can we determine that?
Yes -- use spectra of the system to measure the Doppler shift of each star's absorption lines. If the orbit is circular, then the maximum radial velocity we measure for each star is exactly the same as the tangential velocities VA and VB with which they pass in front of each other.
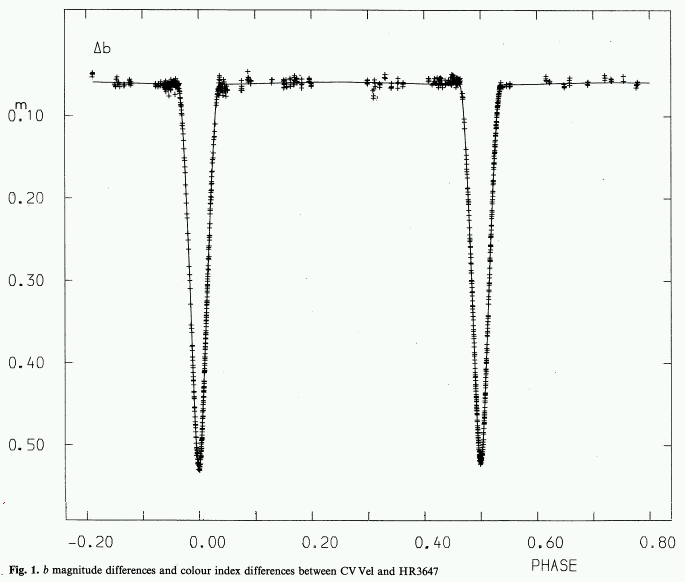
The star CV Velorum, or CV Vel for short, is an eclipsing binary which shows features from both stars in its spectra. The period of its light curve is P = 6.889 days. Here's a sample of its light curve, from the paper Four-colour photometry of eclipsing binaries. VIII - CV Velorum, light curves, photometric elements and absolute dimensions
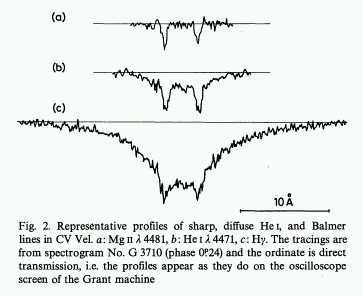
Here are a few small segments of its spectrum, taken at a time ("quadrature") when the components were moving nearly directly towards us and away from us, from the paper Spectroscopic observations of eclipsing binaries. III - Definitive orbits and effects of line blending in CV Velorum In this system, the two stars have nearly the same mass, so they orbit their center of mass with nearly the same velocity. The features you see in the spectrum below are shifted by equal amounts away from the rest wavelength of each line, which is halfway between the features.
Q: What are the orbital speeds of the
primary and secondary stars?
Q: What is the duration of the primary
eclipse?
Q: Assume that the two stars are the
same size. What is the diameter of
each one?
A topic for another day.
So, just how many stars have well-measured diameters? One estimate comes from the Catalogue of Apparent Diameters and Absolute Radii of Stars (CADARS) - Third edition -Comments and statistics. You can query this catalogue using the Vizier facility. The catalog contains diameters for over 9700 stars. Is it a fair sample of stellar sizes? Take a look at the distribution of measured sizes, in terms of the solar diameter:
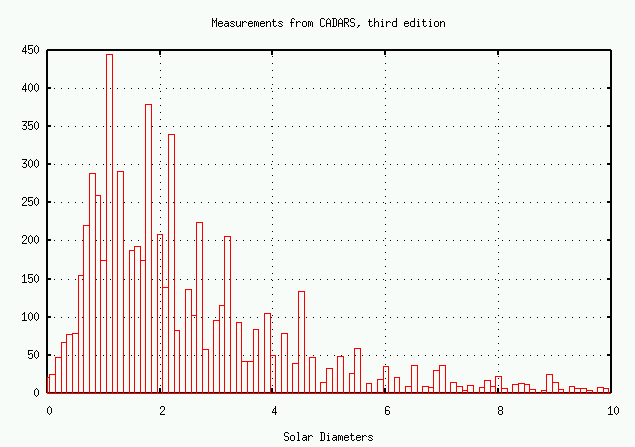
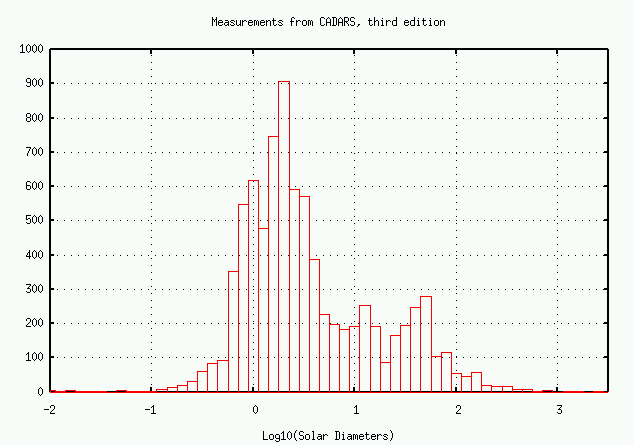
What fraction of these stars are smaller than the Sun? What fraction of all stars in the galaxy are smaller than the Sun? There is clearly a bias towards large stars -- why?
Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.