A really SMALL rollercoaster

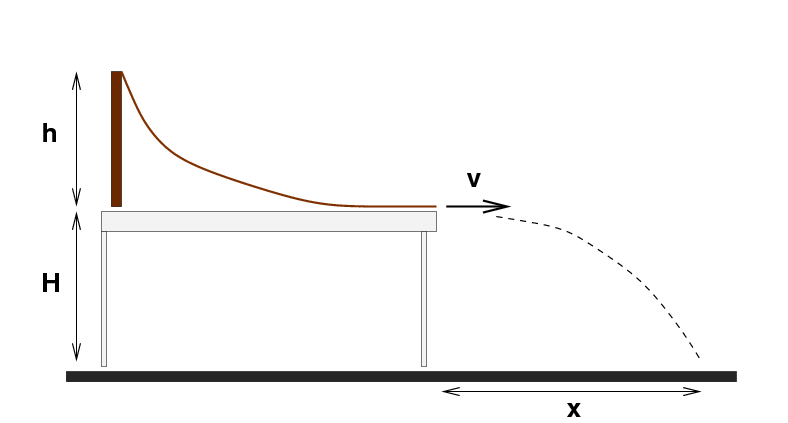
Let's build our own very small roller coaster here in the classroom. I'll set up a tower of height h above the tabletop. A car of mass m will roll down the track from the tower to the edge of the table, then fly outwards with some initial horizontal velocity v. As it falls a distance H to the floor, the car will cover a horizontal distance x.

First, compute some quantities in general: use symbols only, no numbers.

1. Write an expression for the kinetic energy, potential energy, and total energy of the car when it is motionless at the top of the track. What is the origin of your coordinate system? What is the zeropoint of your potential energy?
2. Write an expression for the kinetic energy, potential energy, and total energy of the car when it is about to fly off the table.
3. What will the car's velocity v be when it flies off the table?
4. How far x will the car move horizontally as it falls to the floor?
5. Does this distance depend on the mass of the car?
6. Does this distance depend on the value g, the local acceleration due to gravity?

Now, I'll give you the quantities for the actual equipment in our example; that is, the height h, etc.

6. What is the car's total energy?
7. What will the car's velocity v be when it flies off the table?
8. How far x will the car move horizontally as it falls to the floor?

At this point, we will run several trials to measure the actual distance the car flies after running down the track. I'll write down the results on the board for everyone to see.

If we have time ...

10. Did the actual distance(s) match your predicted distance? If not, why not?

Copyright © Michael Richmond. This work is licensed under a Creative Commons License.