 Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.


Here's one example of the difference a polarizing filter can make:

Image courtesy of
blueshark
Take a look at a pair of images, one taken through a polarizing filter, the other without any filter.

Images courtesy of
the Robotic Antarctic Meteorite Search project

Example: You and your friend Joe are wearing polarizing sunglasses. As you look out on a sunny day, the sunlight is so bright, it nearly blinds you. "Argh," you cry, "if only I could cut this light down to 10% of its current level -- THEN my eyes would be comfortable." "No problem," says Joe. "Here -- take my sunglasses and hold them in front of yours. If we just rotate my pair by the right angle, the light reaching your eyes will drop to 10% of its current level."
Q: By what angle should Joe rotate
his sunglasses as he holds them
in front of your eyes?
(and is this really going to work
very well in practice?)

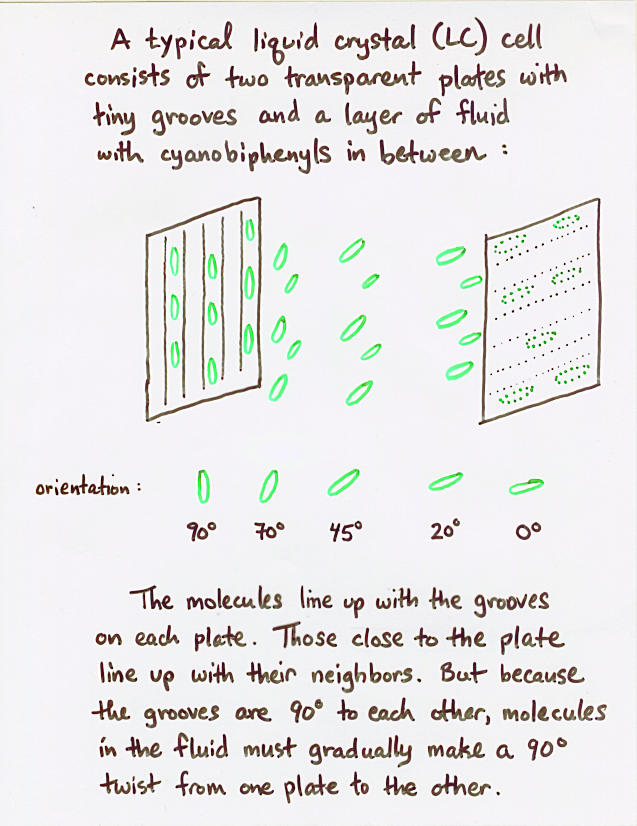
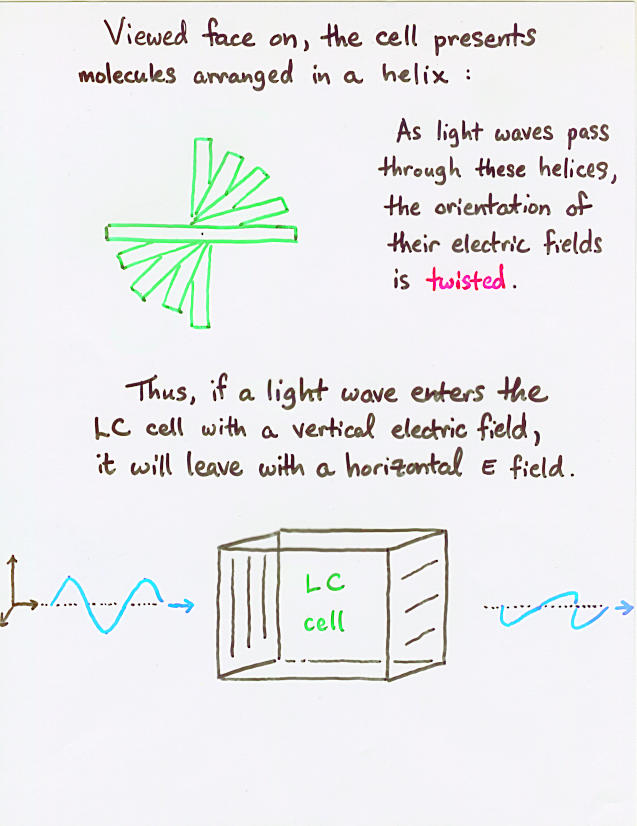
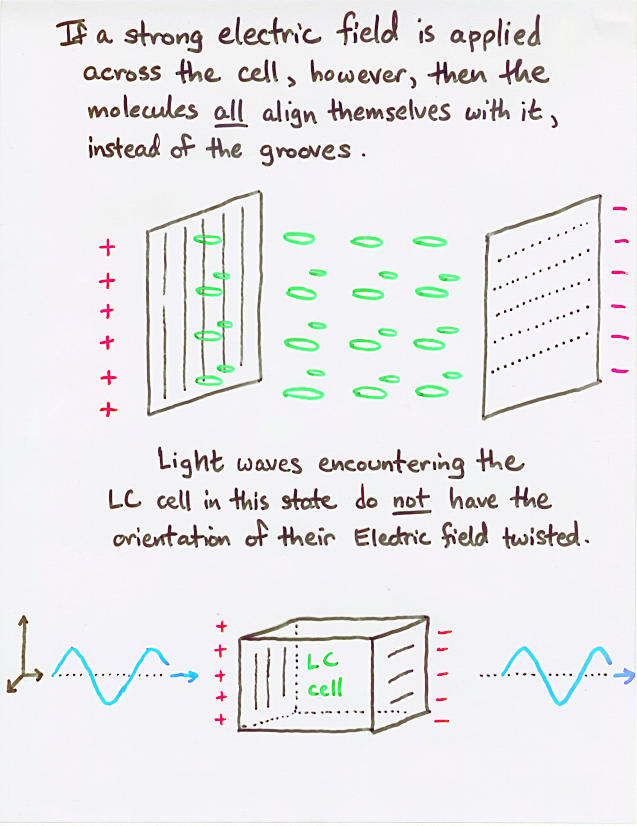
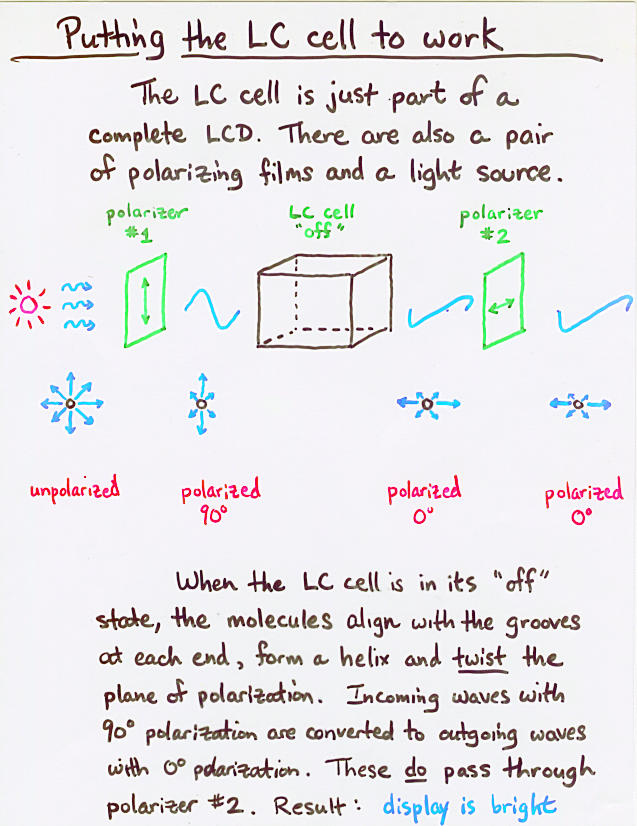
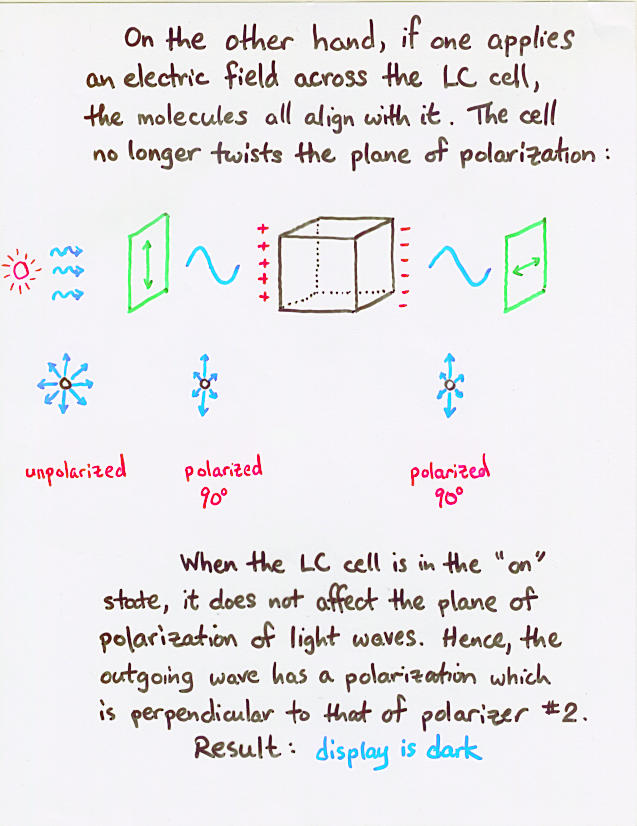


![]()

 Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.