 Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
The Luge track
At the 2010 Vancouver games, competitors are sliding
down a track built in Whistler, British Columbia.
Click on the photo for a picture showing the track's
path clearly.

Some details of the luge course on this track
(I've made a few approximations, since the best
numbers refer to the bobsled course).
Length: 1,374 m
Finish Altitude: 786 m
Start Altitude: 929 m
Number of Curves: 16
Suppose that an athlete of mass 70 kg
slides down the track on top of his
sled of mass 23 kg.
- Under ideal conditions, what is the maximum speed this rider could reach
at the end of the track?
- How much work does gravity do on the rider+sled during the race?
- The actual top speed of the sleds at the bottom
of the course is about
v = 40 m/s.
How much work has friction done on the rider+sled?
- Estimate the coefficient of kinetic friction
between sled and ice.
Here is a closeup of the final turn:
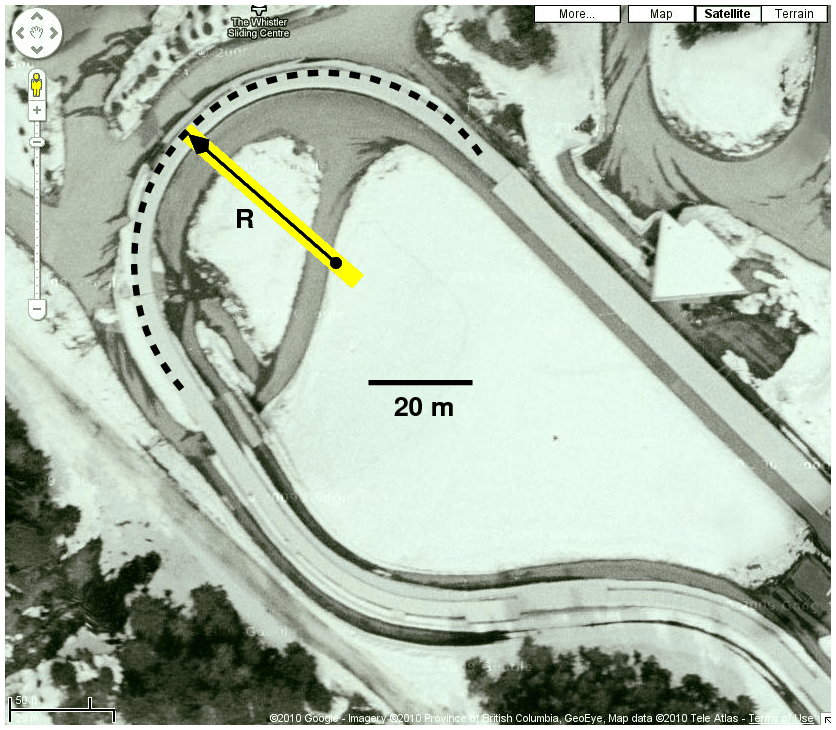
- Suppose the actual speed at the finish line is about
40 m/s.
When a sled moving at that speed goes around
the final turn, what is its
centripetal acceleration?
Express your answer in m/s^2 and in gees.
- What is the angular speed of the rider through this turn?
Provide a magnitude, direction, and units.
- What is the torque exerted on the rider+sled,
measured around the center of the turn,
as they zoom around?
Provide a magnitude, direction, and units.
For more information
 Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.

 Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.

 Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.