




 Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
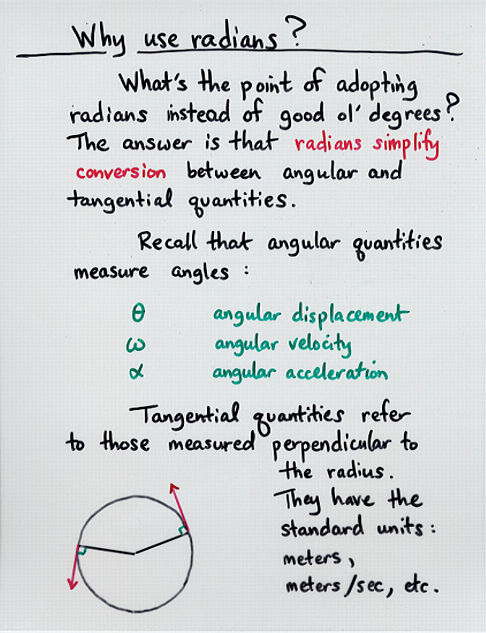
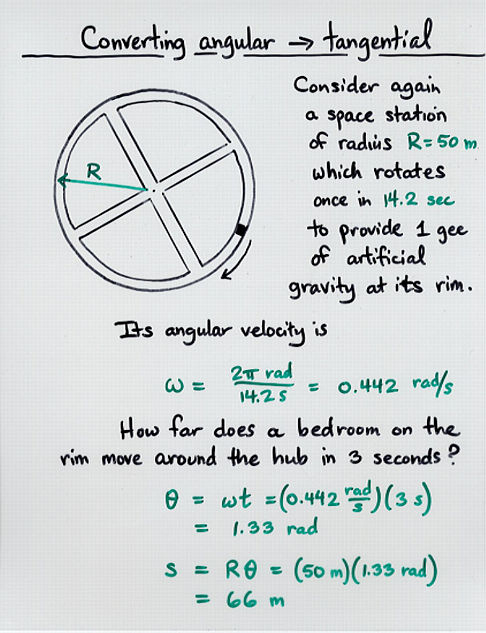
tangential displacement s = R * omega * t
tangential speed v = R * omega
T
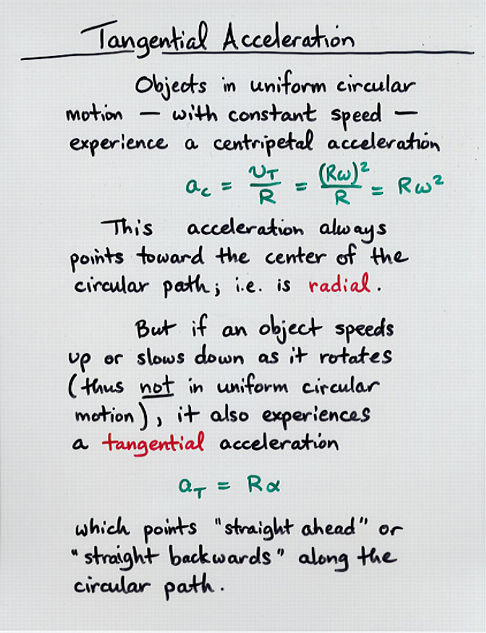
tangential acceleration a = R * alpha
v^2
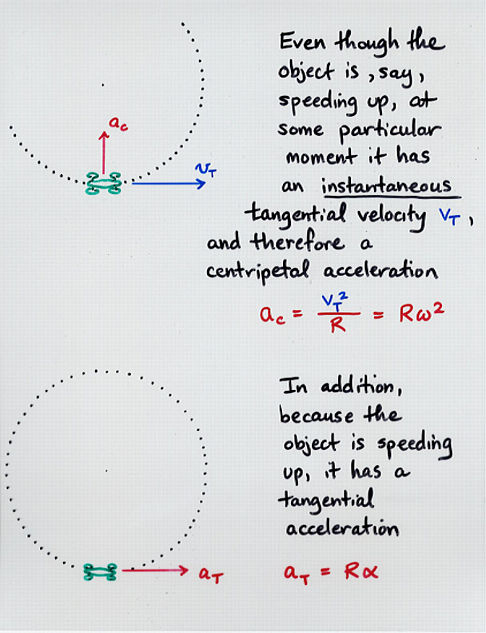
centripetal acceleration a = -----
c R
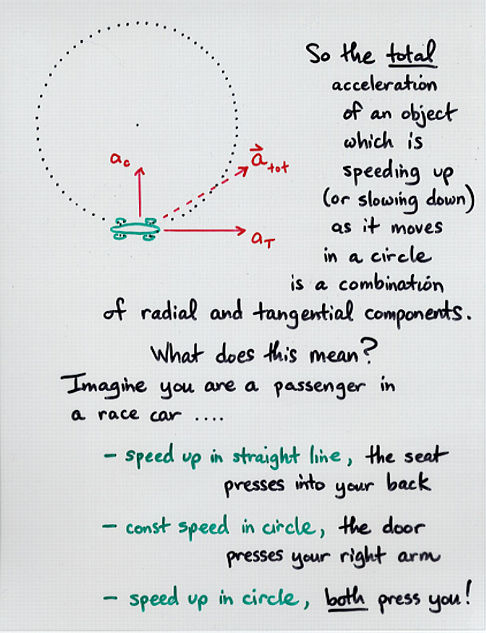
But it also has a tangential acceleration due to its change
in tangential speed; one way to express it is
tangential accleration a = R * alpha
T










 Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.