
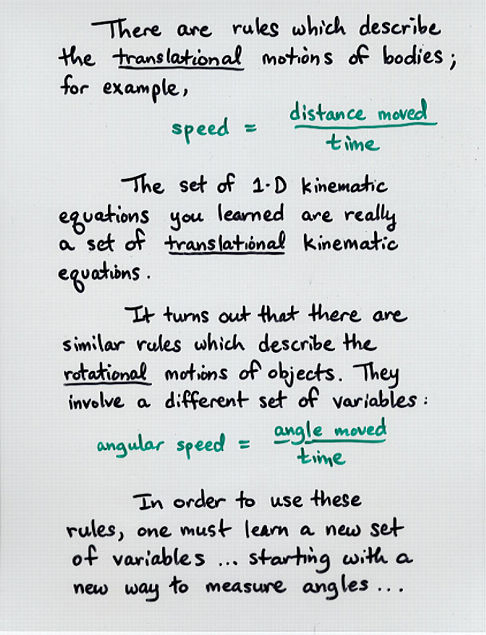


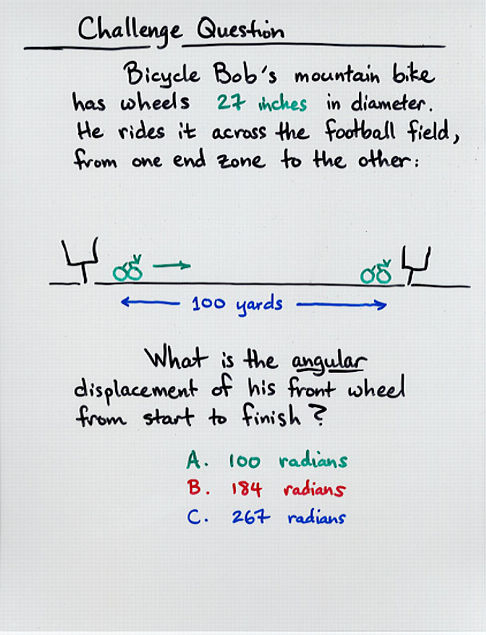



 Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
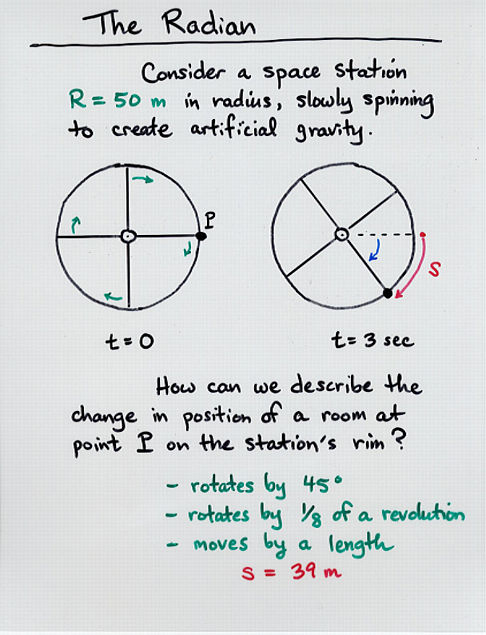
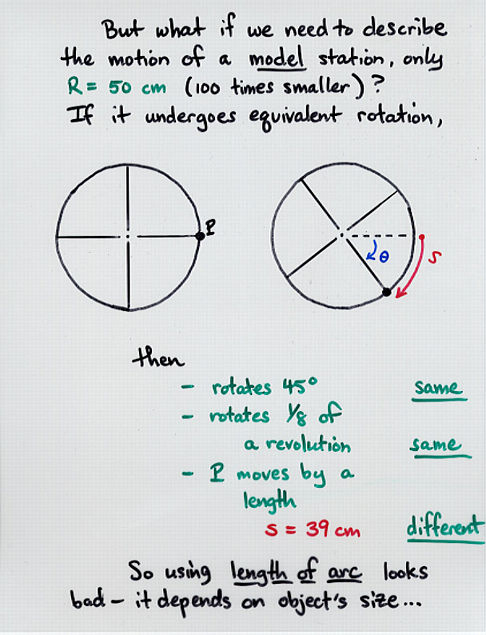
length of arc
angle in radians = ---------------
radius
There are 2*pi = 6.28 radians in one full revolution.
Therefore
2 * pi radians = 360 degrees
--> 1 radian = 57.3 degrees
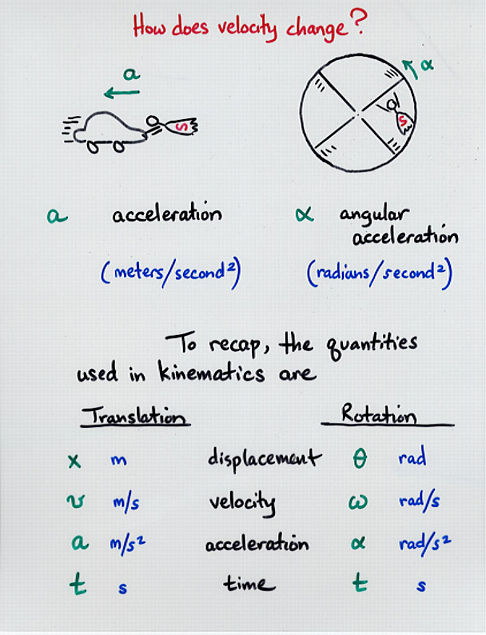
displacement (meters) --> angular displacement (radians)
velocity (meter/sec) --> angular velocity (radian/sec)
acceleration (meter/sec^2) --> angular acceleraion (radian/sec^2)











 Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.