 Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
Center of Gravity and Newton's Second Law in Rotation
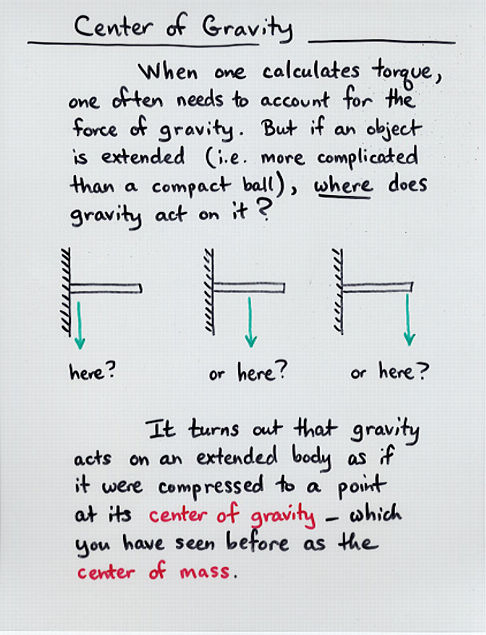
- The center of gravity of an object is the point
one can use as the place where gravity pulls on the object.
That is, when calculating the torque due to gravity on
an extended object, one can pretend that the entire
gravitational force acts only at that point.
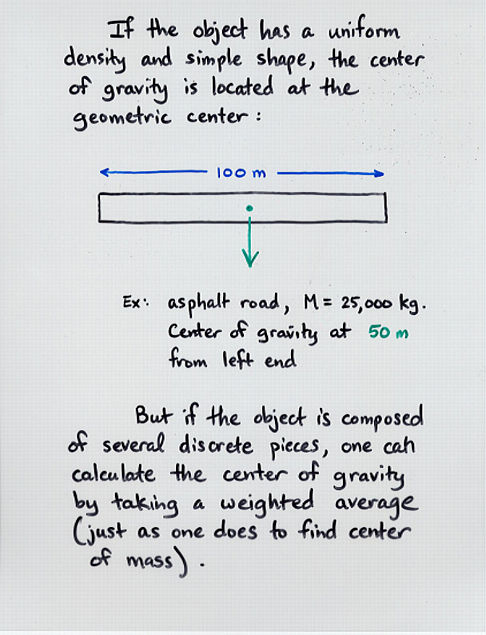
- The center of gravity is exactly the same as the center of mass.
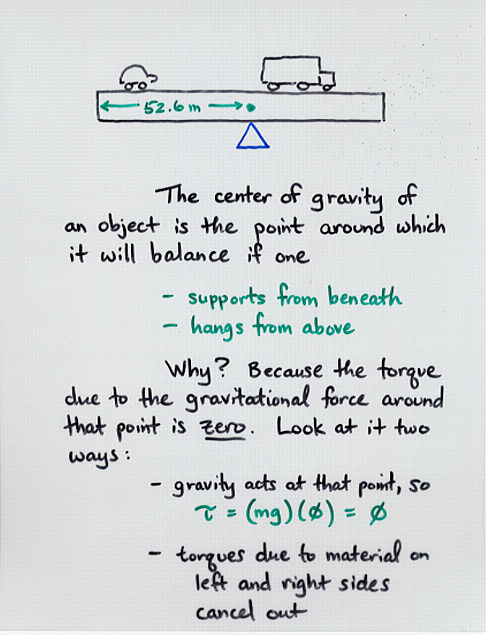
- Objects balance if supported beneath their center of gravity
(which can sometimes help one to find it).
- Newton's Second Law in translational motion looks like this:
force = (mass) * (acceleration)
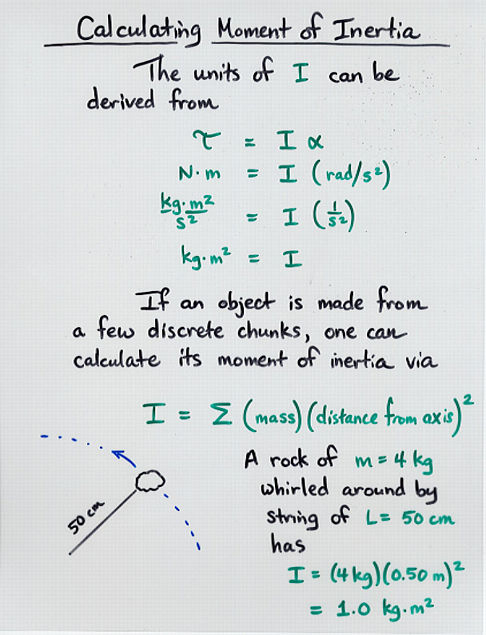
The rotational analog to it is
torque = (moment of inertia) * (angular acceleration)
- Moment of inertia depends not only on the mass of an
object, but also its distribution: material far from the axis
of rotation adds more rotational inertia than material close
to the axis.
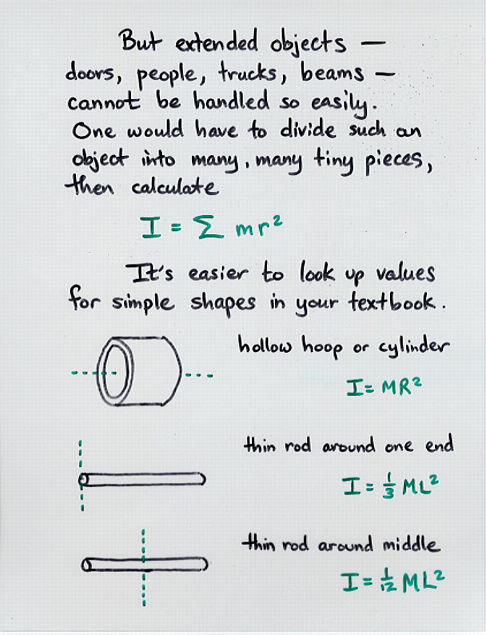
- One can calculate I, the moment of inertia, for simple
object as
2
I = Sum of (mass) * (distance from axis)
- For extended objects, it's usually best to look up values
of moment of inertia in your textbook. See p. 253 in
Cutnell and Johnson.
- The moment of inertia has units kg * m^2.
Viewgraphs
 Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.



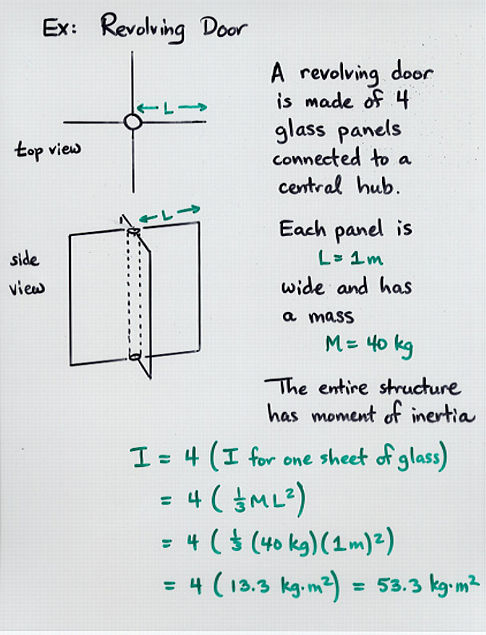
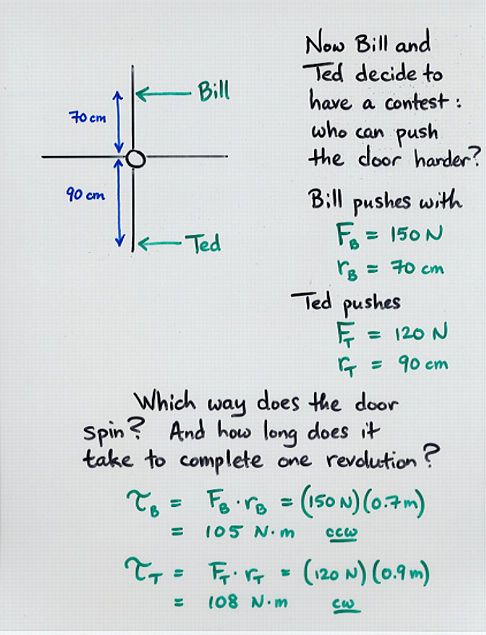

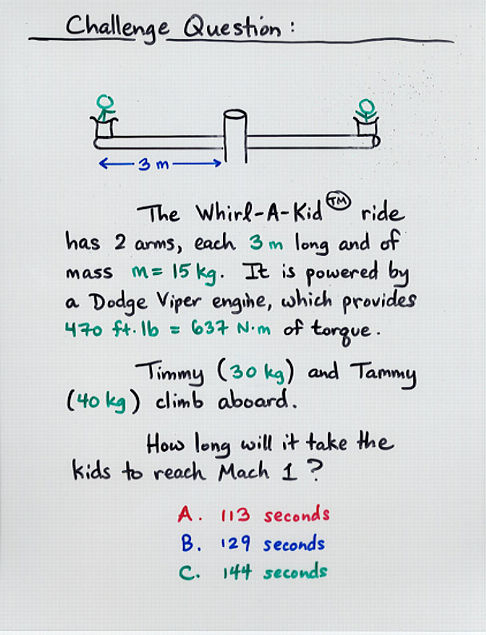
 Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.












 Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.