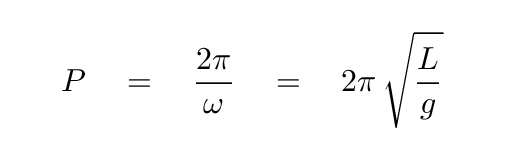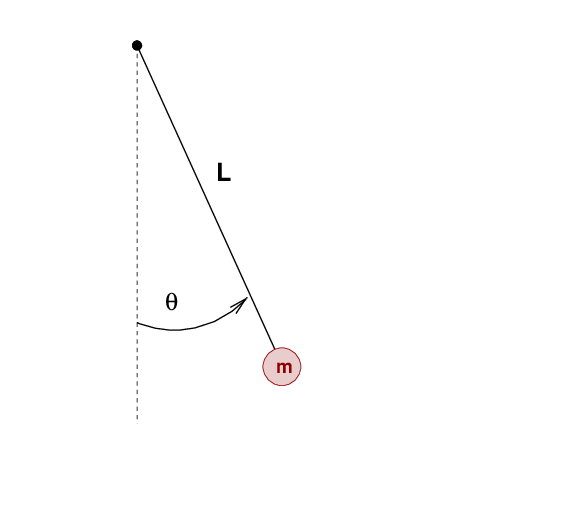
What causes a pendulum to swing back and forth, back and forth, in such a regular manner? Let's take a look at the TORQUES acting on the bob of a simple pendulum.
Suppose that the bob, of mass m, hangs from a massless string of length L. We displace the bob by an angular displacement θ, then release it.

Q: What are the forces acting on the pendulum bob?
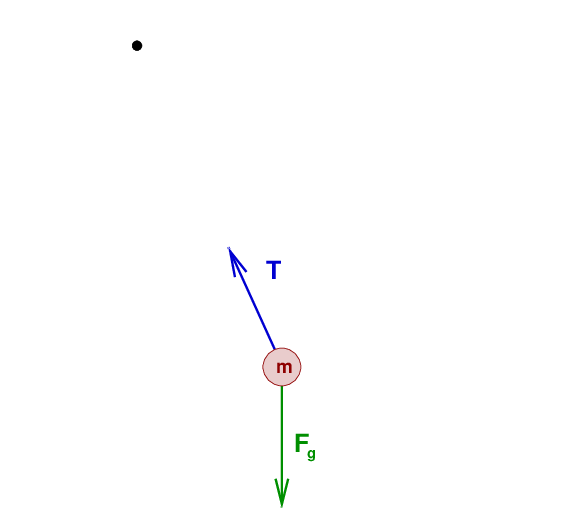
Okay, so there are two forces. We know the bob will swing around the pivot at the top of the string, so let's compute the torque around this point due to each force.
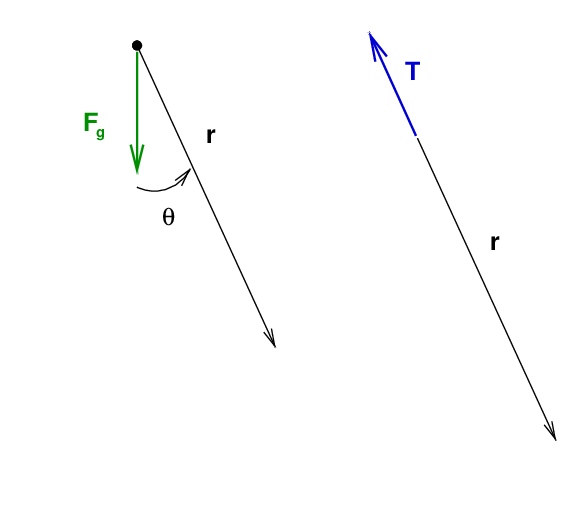
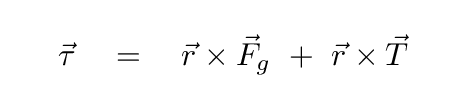
Hmmm. The radius vector and the tension force are exactly 180 degrees away from each other, so their cross product is zero. The only torque is due to the force of gravity.
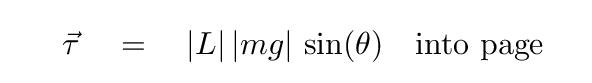
Now, if we limit the motion of the pendulum to SMALL ANGLES, no larger than, say, 0.10 radians, then we can make the small angle approximation: sin(θ) ≈ θ. Thus,

Look at the picture again. In which direction is the angular displacement θ?

Q: In which direction is the angular displacement θ? Q: In which direction is the torque τ?
Right. The displacement is OUT of the page, but the torque goes back INTO the page. Since the two vectors point in opposite directions, we can write
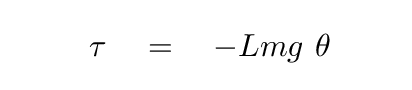
Okay. At this point, we can use the fact that Newton's Second Law in rotational motion states that the torque on an object is equal to its moment of inertia times its angular acceleration.
Q: What is the moment of inertia of the pendulum bob around the pivot?
Right.

Therefore,
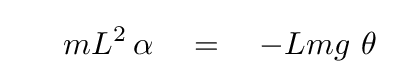
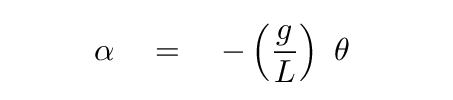
Of course, the angular acceleration is just the second derivative of angular displacement with respect to time.

Aha! That's a differential equation that we've seen before. We know how to solve it -- the solution will involve sines or cosines. To make the result look nicer, let's define a variable
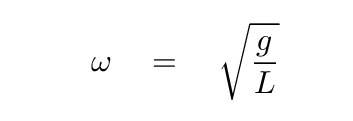
so that we can write the differential equation as
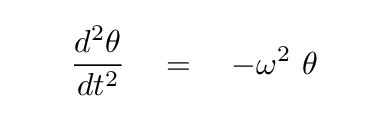
Q: What is the solution to this differential equation?
Can you write an equation for the angular position
of the pendulum as a function of time?

In this equation, the amplitude A is the maximum angular displacement from the vertical position.
Most people don't measure the angular frequency ω of a pendulum directly. Instead, we usually measure its PERIOD -- the time it takes to complete one full swing, there and back.
Q: Can you write the period of a pendulum in terms of
its length and other variables?