Water now flows very slowly through the river bed, covering all but the tips of the islands. The water moves at speed u. You can't walk across the river any more, but must instead swim. Fortunately, you were on the Olympic swim team, and can swim at the same speed v that you normally walk.

When you try to swim directly across the river, you feel the current pulling you downstream. In order to move straight across from one bank to the other, you have to swim at an angle, pointed slightly upstream.

In fact, you have to point yourself just as shown in the figure at right.

What is the horizontal side of the triangle formed by these components of velocity?

____________________

Suppose that the water flows at u = 0.1 m/s, and you swim relative to the water at v = 1 m/s. What is the horizontal component of your speed?

____________________

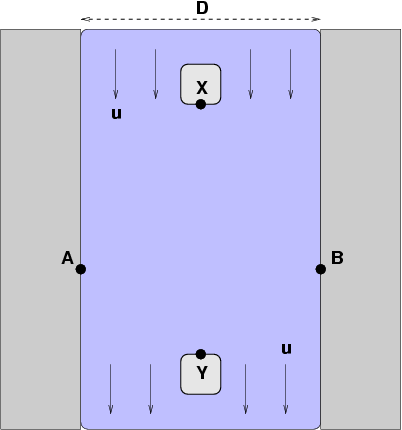
Now, you aim your body upstream slightly and swim so that you go directly from A to B. How long does that trip take? Write down the algebraic expression, not the actual number.

____________________

____________________

____________________

On the other hand, suppose you start on island X and swim downstream to the other island, Y. You go with the current. How long will that take?

____________________

____________________

____________________

Now you can plug in some numbers: again use D = 2 km, v = 1 m/s, and u = 0.1 m/s. What are the times required for the two round trips?

____________________ vs. ________________